I’ve always been attracted to vanishing points, so I’ll discuss them a little bit and describe how we experience a similar type of phenomenon sonically everyday.

Here’s a photo I took a couple of weeks ago while thinking about this post. I was looking for the quintessential vanishing point and think that I might just have succeeded!

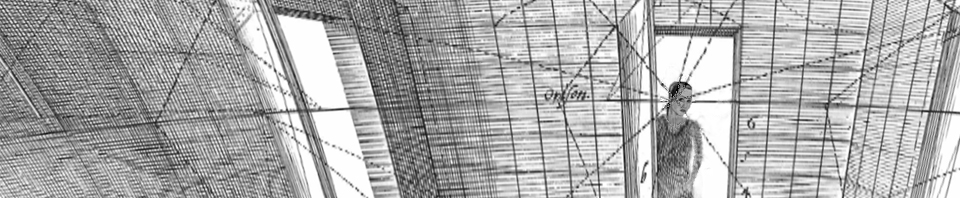
Another photo I took a few years ago inside the abandoned Silo no 5 in Old Montreal. For more photos and info on a cool sound installation by the user inside that same building, check out Silophone (2000).

From the above photos, it’s also probably becoming evident that I’m attracted to urban decay. I’m sure there will be more to come on that subject, but back to the one at hand.

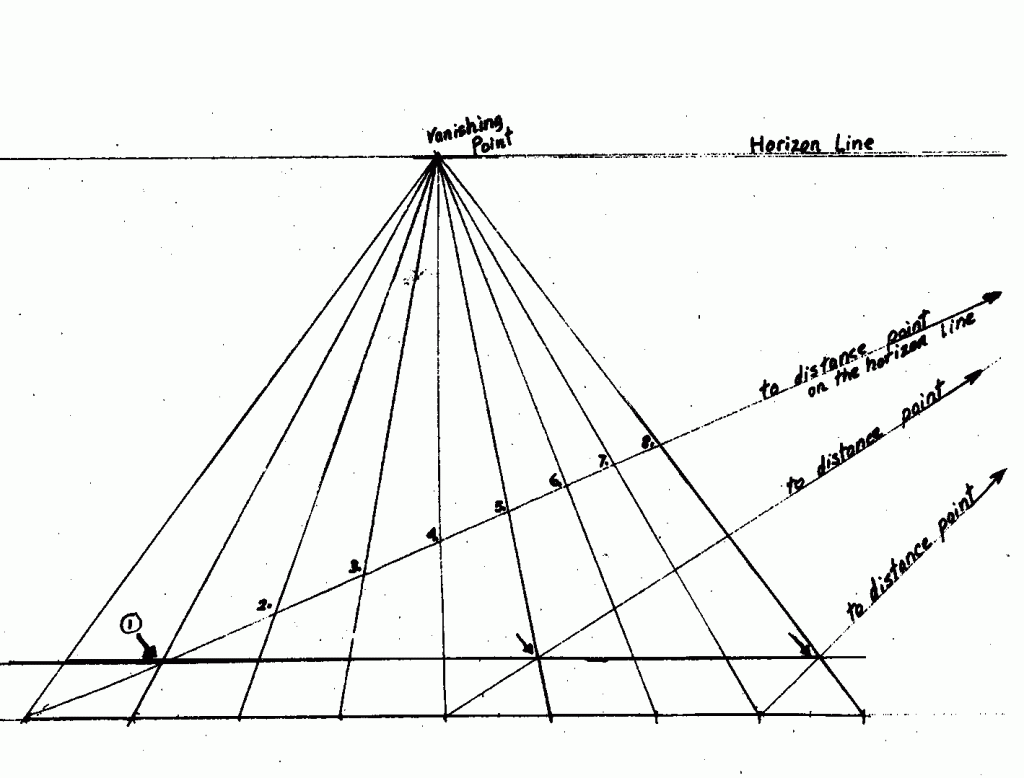
A vanishing point is a point in the distance on the horizon towards which the orthogonal lines (which are otherwise parallel) converge when going from foreground to background.

We know that objects are smaller when further away, so then is the distance between objects which is why these lines must converge. In the above cases, we’re looking at a one-point perspective meaning there’s only one vanishing point and we are facing it directly. In the photos, the tracks and conveyer belt (which form the orthogonal lines) are converging on the vanishing point where the lines going in the other directions (from the left to the right or the floor to the ceiling) remain parallel.

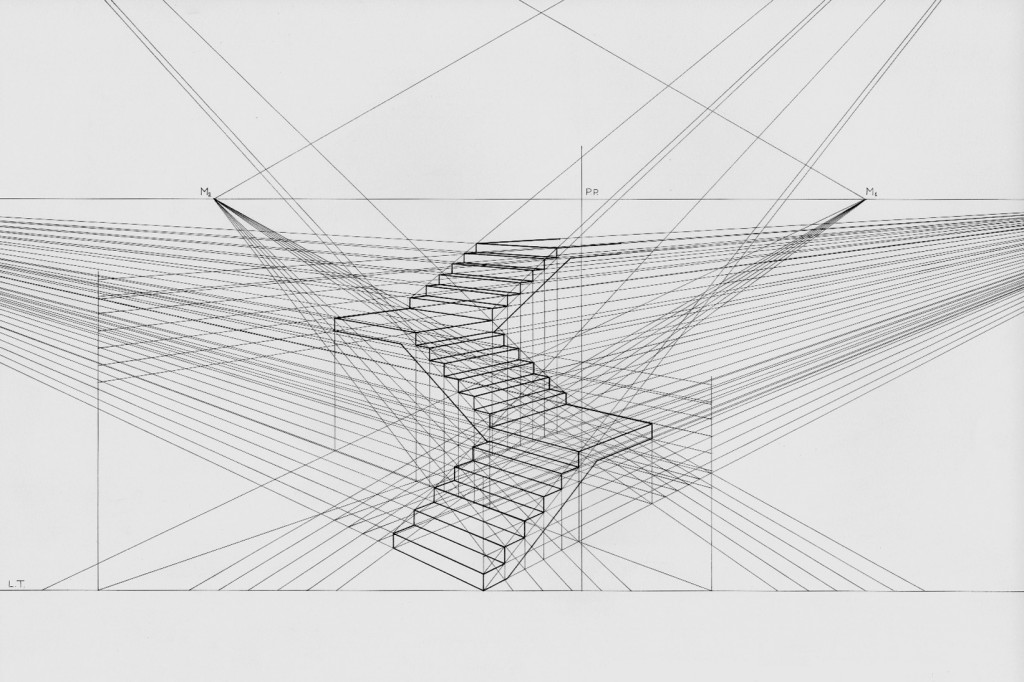
Here’s a drawing that has a two-point perspective. We’re looking at the staircase at eye-level but at an angle, so there are vanishing points heading diagonally back towards the left and the right:

Looking from above or below something, we can get a three-point perspective.

Here vanishing points are heading back diagonally to the left and right side, but there is a new vanishing point up above.

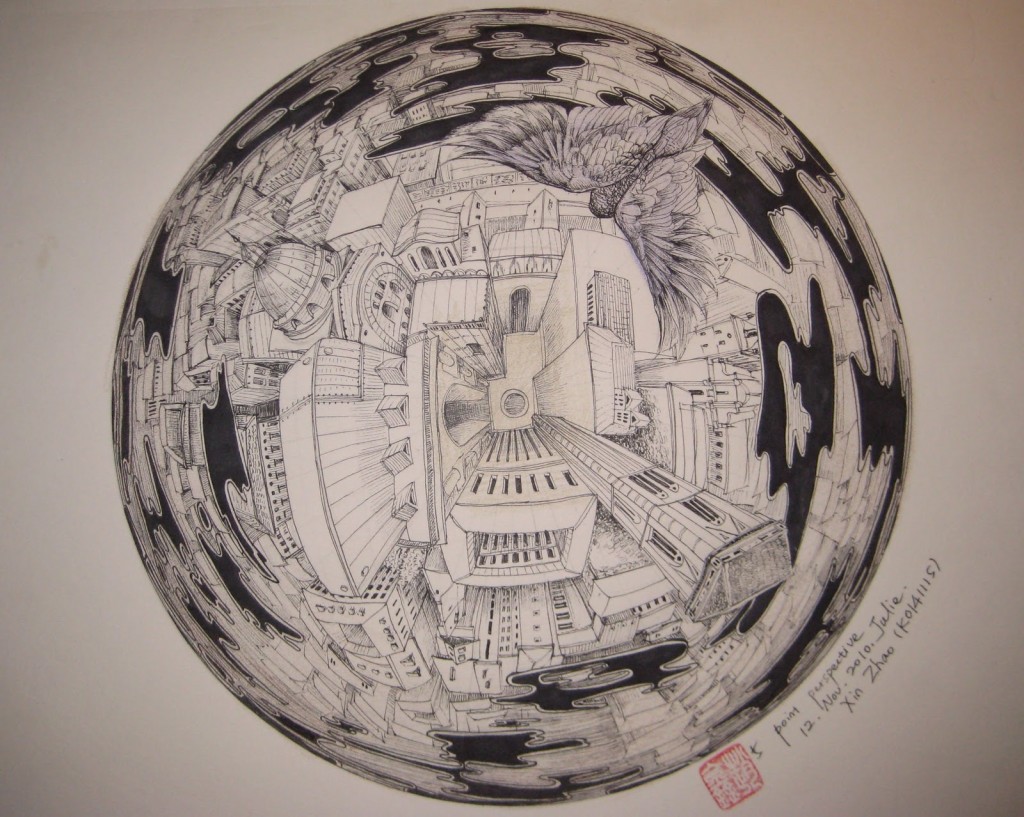
Julie Zhao – 3 Point Perspective

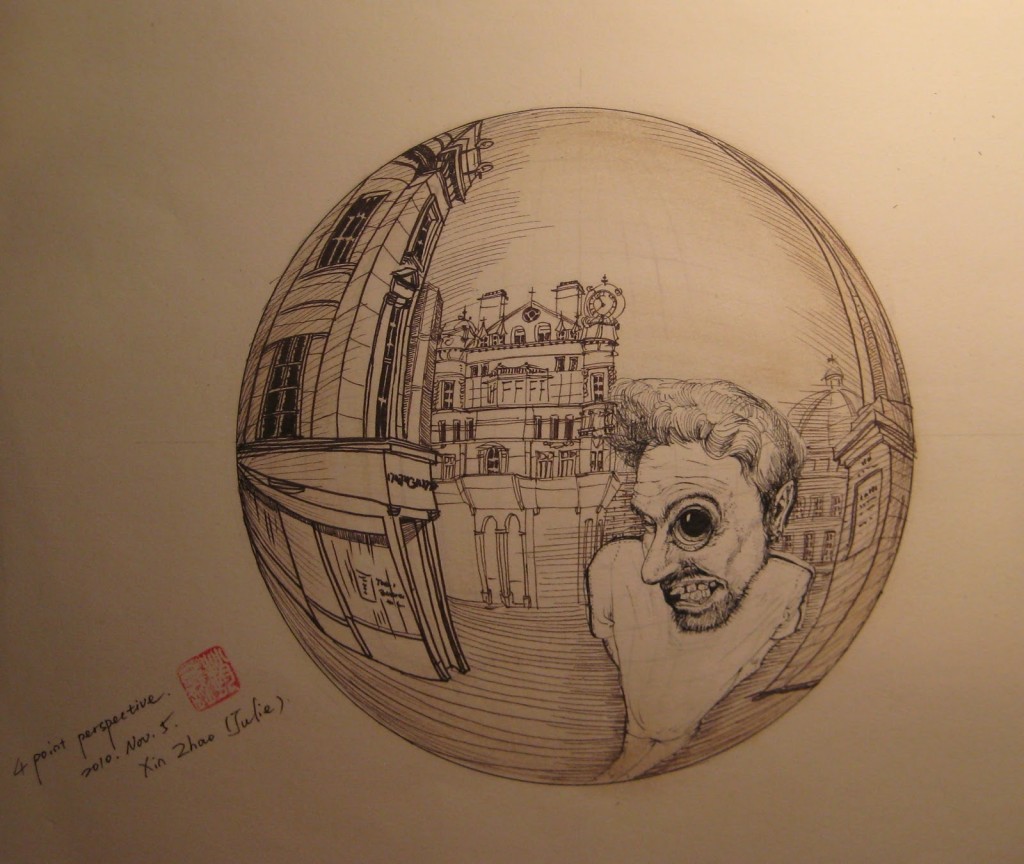
A 4 point perspective contains the same vanishing points as the 3 point perspective above with an additional one heading down towards the ground.

Continuing with examples from Julie’s work, here’s her 4 point perspective.

And my favourite, her 5-point perspective – breaking into perspective 3D, we have our 4 point perspective receding in each direction off a plane plus our new Z axis (altitude) forging its way into depth.

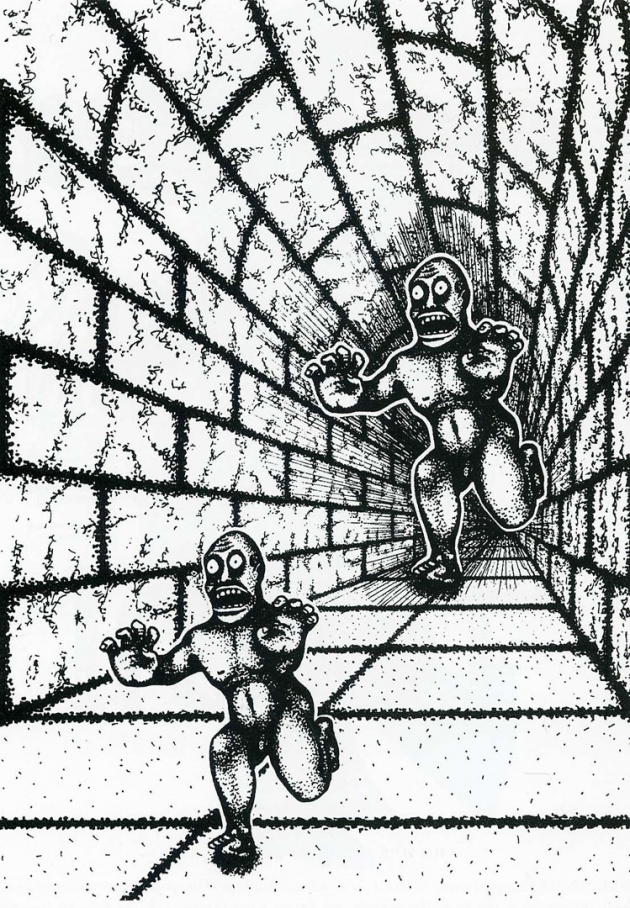
Let’s simplify a little and head in another direction. Roger Shepard took only partial cues from one-point perspective and had a bit of fun. The vanishing point is there, but the figure’s size remains constant making it seem like a figure is being chased by a giant version of itself.

Roger Shepard – Running Monster (from Terror Subterra)

Now, let’s play around with relativity for a moment. In the above picture our eyes tell us that we have one large figure and one small figure running through a tunnel. Looking at it from a different perspective (probably the artist’s perspective), we have two figures that are the same size running within a distorted tunnel that is deceptively mimicking a vanishing point. (check out this interactive version)

It’s a very similar idea to the amazing kitchen scene from Michel Gondry’s Eternal Sunshine of a Spotless Mind:

This is also achieved through a play with the vanishing point. Clearly, objects are not getting smaller as they get further away from the camera. The vanishing point seems to have been inverted and is in fact on our side of the screen and not in the distance (called reverse perspective). The entire kitchen expands on orthogonal lines away from that point allowing the kitchen table (and everything else but Michel himself) to get larger the further you go back while maintaining appearances. This isn’t really a camera trick in the end but a much more labor intensive feat of set design. The camera’s position though is integral to the success of the effect; moving it away from the reversed vanishing point would quickly throw the perspective and alert us that something fishy is going on. Reverse perspective has also been used to different ends in Byzantine and Russian Orthodox figures, as well as in cubism and other modem art. It’s theorized that this reversed perspective was originally meant to show that the subject is looking upon you or that the subject is omnipresent. A new spin on Eternal Sunshine?

How does this all relate to sound?

Well, for starters both our eyes and ears work together in stereo (visually or phonically) and, singled out, each eye or ear has a single point (or mono) perspective on the world. If you close one eye, depth is still hinted at in the relativity of objects and lighting cues like shadows, but it’s definitely more difficult to perceive than when using both eyes. Similarly, with one ear you might be able to gain clues within a space, using direct and indirect sounds, their timings and relative amplitudes, but the full image is much more apparent when you’re using both ears. The obvious difference between the two senses is that your ears are on either side of your head where your eyes are facing the same direction (interestingly because we are predatorial).

Given these physical similarities, though, are there then sonic vanishing points? Despite any trickery, vanishing points will always extend away from your eyes (or if you’re looking at an image, your transposed eyes). The point is so far away that anyone standing there would be reduced to a small indecipherable dot, because, as we know, as something visual recedes into the distance it gets smaller. We can start by drawing a comparison between an object receding visually and sonically.

A brief interlude:

Let’s say that Nicolas Cage is freaking out in front of you and, as he’s doing so, he’s walking backwards away from you. As he recedes, he’ll appear increasingly smaller and the amplitude of his freak out, as well as certain frequencies in his voice that don’t carry as well, will diminish. Visually and sonically then, he’s getting smaller.

There’s one thing missing though. Visually, a vanishing point recedes into the distance while drawing orthogonal lines into it. In other words, our example is better with not just one Nicolas Cage freaking out (one orthogonal line) but two or more! For your safety, let’s say just two Nicolas Cages are freaking out as they’re walking backwards away from you. To be more precise, say they start on either side of you and walk backwards along parallel lines a few feet apart (like railway tracks). Now we’re really getting somewhere, we have a sonic version of train tracks!

So what happens?

Well, we already know that the amplitude of their voices and the frequency content within them will diminish as they get further and further away from us, but something else will happen as well. When they are close to you, you’ll be able to clearly distinguish the Nicolas Cage’s freakout on your left from the one on the right. As they move back however, and despite the fact that they are moving along parallel lines, they will move stereophonically towards the centre. Here’s what it looks like:

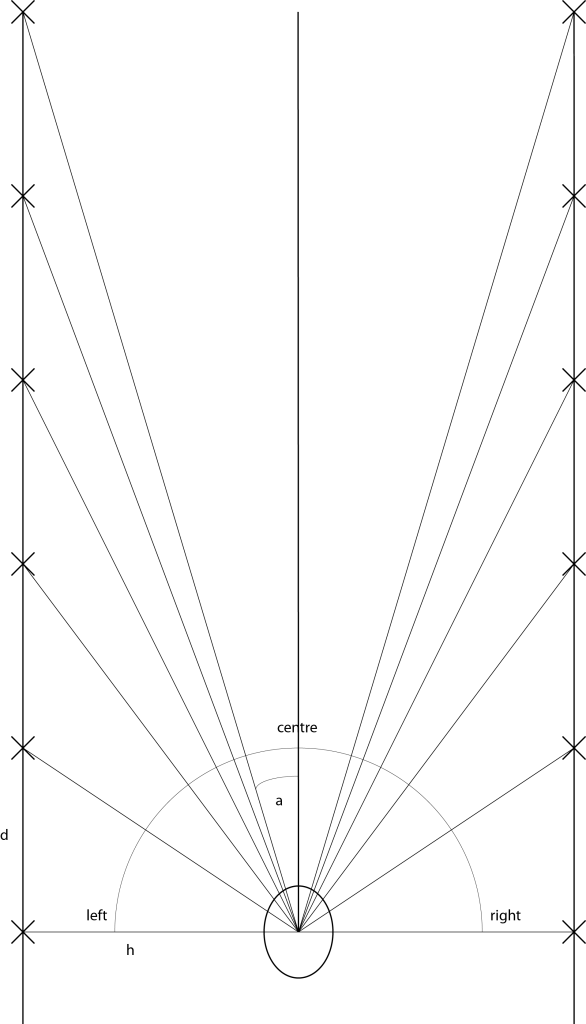
As the X’s on each side get further, the direct path of sound converges on a line that extends straight out in front of you. For those of you more mathematically oriented:

<math>

Where h is the distance from your head to the track, d is the distance travelled along the track and a is the angle of the sound’s path from stereo centre right in front of you…

a = tan-1 (h/d)

As d gets larger (or as the sound moves further down the track), h/d will get smaller since h is constant. As h/d approaches zero angle a will approach 0 degrees, which is equivalent in this case to centre on our stereo spectrum.

</math>

So, in the same way that visually parallel lines come together towards a central point in the distance (the vanishing point), so does sound move stereophonically towards a central point as it moves away from you.

And that, my friends, is a sonic vanishing point!

To end the post, here’s a beautiful photograph from Eugene Richards (I think it’s from him anyway):